To... boundlessness and... above?
I'm talking of course about the infamous catchphrase of Buzz Lightyear in Toy Story or, as Imdb puts it:
What is the meaning of "To infinity and beyond?"
It seems a throwaway line, but hidden there is a deep philosophical meaning. It pops up here and there in pop culture and even in random discussions with friends. In 2001: A Space Odissey just before the psychedelic "Star Gate" sequence a title card appear, "Jupiter and Beyond the Infinite" (an inspiration for Buzz?). In the hit Single Ladies (Put a Ring on It), Beyoncé sings "... and delivers me to a destiny, to infinity and beyond.", There are then the album Beyond the Infinite, by trance group Juno Reactor, or the Greek film Eternity and a Day, by director Theo Angelopoulos. In 2008 this sentence helped father and son to survive a shipwreck. And let's not forget infinity plus one! And the lovey-dovey couples smooching "I love you infinity times and more". There are probably infinite other examples (and more!).
It sounds paradoxical: how can you go beyond the infinity? It's not possible to reach the end of infinity (because it is infinite), so how can one overtake it? One needs to change completely point of view: from potential infinity to actual infinity.
Let me go back to the example of Achilles and the tortoise, just like this blog started. You have the tortoise and Achilles, say, 10 meters apart, Achilles in the back. They run. Achilles is much faster than the tortoise, so when Achilles reached the point where the tortoise was, the tortoise just made one meter. Achilles run that meter, and the tortoise is 10 cm ahead. And so on, and so on. Will Achilles ever reach the tortoise?
If you have the mentality above, it will not: you have this infinite succession of states: 10 m distance, 1 m distance, 10 cm distance, ... and you cannot reach the end of it, because it is infinite! This is the so-called potential infinite, potential because you never realize it in full, you just see parts of it.
But we know that Achilles reaches the tortoise, right? We're not that stupid. So what? Well, this is where Cantor shines! He managed to treat in a formal way the actual infinite. You pick all the infinity, you put it in a sack, and you treat it as a whole, completed object. So you can imagine what happens after it, in this case Achilles overtake the tortoise, turns and makes very funny faces. "Brlbrblrblrl".
Think of the potential infinity as an infinity you are walking inside. Like, just go out now and try to reach the horizon, it will always go on and on and you will never reach it. But the actual infinity is like seeing everything from above, so instead of walking pick a drone and go up, up, up, and you can embrace everything with a single view. (Of course, the metaphor works only if our Earth is flat and infinite, sorry, so I guess that makes no sense).
Another Zeno's Paradox: the dichotomy paradox. You have to go to a bus station that is 1 km far. To go there, you have to go half kilometer far. And then a quarter kilometer more. And then an eighth more. How can you reach the bus station? In mathematics, the distance you make is written like this:
According to a potentialist, this makes a potential 1, that is, is closer and closer to 1 without ever reaching it. According to an actualist, this is 1. What is the difference, you say? Look at this:
How much is this? According to an actualist, it is 2. According to a potentialist, it is BLAARGH GET THAT AWAY FROM MY FACE! SATAN!
Let me go back to the example of Achilles and the tortoise, just like this blog started. You have the tortoise and Achilles, say, 10 meters apart, Achilles in the back. They run. Achilles is much faster than the tortoise, so when Achilles reached the point where the tortoise was, the tortoise just made one meter. Achilles run that meter, and the tortoise is 10 cm ahead. And so on, and so on. Will Achilles ever reach the tortoise?
If you have the mentality above, it will not: you have this infinite succession of states: 10 m distance, 1 m distance, 10 cm distance, ... and you cannot reach the end of it, because it is infinite! This is the so-called potential infinite, potential because you never realize it in full, you just see parts of it.
But we know that Achilles reaches the tortoise, right? We're not that stupid. So what? Well, this is where Cantor shines! He managed to treat in a formal way the actual infinite. You pick all the infinity, you put it in a sack, and you treat it as a whole, completed object. So you can imagine what happens after it, in this case Achilles overtake the tortoise, turns and makes very funny faces. "Brlbrblrblrl".
Think of the potential infinity as an infinity you are walking inside. Like, just go out now and try to reach the horizon, it will always go on and on and you will never reach it. But the actual infinity is like seeing everything from above, so instead of walking pick a drone and go up, up, up, and you can embrace everything with a single view. (Of course, the metaphor works only if our Earth is flat and infinite, sorry, so I guess that makes no sense).
Another Zeno's Paradox: the dichotomy paradox. You have to go to a bus station that is 1 km far. To go there, you have to go half kilometer far. And then a quarter kilometer more. And then an eighth more. How can you reach the bus station? In mathematics, the distance you make is written like this:
According to a potentialist, this makes a potential 1, that is, is closer and closer to 1 without ever reaching it. According to an actualist, this is 1. What is the difference, you say? Look at this:
How much is this? According to an actualist, it is 2. According to a potentialist, it is BLAARGH GET THAT AWAY FROM MY FACE! SATAN!
Artist's rendition
The potentialists say that this is just a trick, that our limited minds just cannot comprehend actual infinities, and yet, mathematics goes just smoother with them, and the paradoxes are solved. Thanks, Cantor!
You know what? Let me see what the Internet thinks that "To infinity and beyond" means...
Oh, boy.
Fun fact: there are many, many mathematicians, even big experts, even Fields medals, that just have no clue about this. They work in mathematics like they were in the 19th century, willfully ignoring that all their work is founded on this.
This is the first results in Google (hopefully the second after I post this).
Definition in Mathematical Circles:
What exactly does Buzz Lightyear mean when he says, "To Infinity and beyond!"? A few professionals at Harvard investigated the origin of this quote and traced it back to limits. According to Dr. Sanjay Gupta, "Buzz Lightyear is a metaphor of a function which approaches a certain number, but never actually reaches it." However, experts at MIT believe that Buzz Lightyear is referring to vertical asymptotes. Dr. Benjamin Hernandez says, "It is possible to cross horizontal asymptotes, but verticals are impossible. Buzz Lightyear is showing everyone that he can do the impossible and cross horizontal and vertical asymptotes."
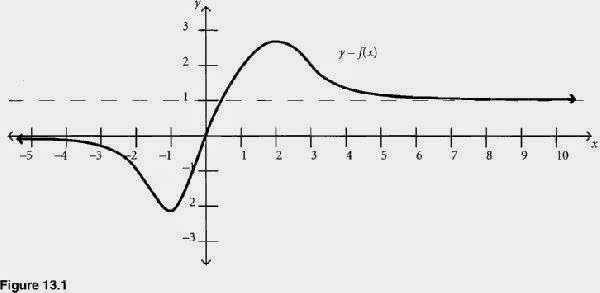
Now, I don't know who are these guys. Most probably they are misquoted, maybe they don't exists, so I am not really against them. But what is written there, trying to sound professional as heck (Harvard! MIT! Bum!) is far-reaching or just nonsense. The first sentence, by Dt Sanjay Gupta, is a typical expression of potential infinity (it approaches a certain number but never actually reaches it) and does not explain anything. Where is the beyond? The second sentence makes more sense: here is a horizontal asymptotes:
The asymptote is that dashed line. See? The function crosses it. Here is a vertical asymptote:
The function doesn't cross the line. And Buzz can! Well, OK, but... isn't this a little bit too technical? Also, isn't it a bit underwhelming that "Infinity and beyond" means a little bump in a line, just like your body when you eat too much Marshmallow Fluff?
The asymptote is that dashed line. See? The function crosses it. Here is a vertical asymptote:
The function doesn't cross the line. And Buzz can! Well, OK, but... isn't this a little bit too technical? Also, isn't it a bit underwhelming that "Infinity and beyond" means a little bump in a line, just like your body when you eat too much Marshmallow Fluff?
INFINITY!
So, forget about what you read online. The meaning of "To infinity and beyond", even mathematically, is: we all think that we are trapped in our human limits, without escape, but in the end it is just an illusion. Buzz (and Cantor) is showing us the way to recognize the illusion, change the perspective, finally break free and go! leaving all our chains behind, going were it was previously unthinkable, unimaginable.
You hear that, potentialists?
PS. I am actually going to Harvard next week. If I meet Dr. Sanjay Gupta, I'll let you know.
Statistics says that probably this is the first page of the blog you are reading. Then, may I suggest to read the manifesto, to understand what is this about? Or just skip to the meaty parts, like the posts on "The Fault in Our Stars", or "The Big Bang Theory", or a cute AT&T advertisement.








The trembling guy, is that how a potentialist feel when he/she is lead to the real of the first limit ordinal? That is a cool thing :D
ReplyDeleteGot chill when reading this I love your outlook.
ReplyDeleteDid you meet Dr. Sanjay Gupta ?
ReplyDeleteHa ha, no, in the end I could find no Sanjay Gupta in the department of mathematics. Maybe he was in another department, bu I felt that going around the campus asking for a guy I've read on the internet would have been embarassing for my host...
ReplyDeleteYes, this is likely a paraphrase of Kubrick, but the Macho Man Randy Savage actually said the phrase exactly as "To infinity and beyond" twice in a promo. Maybe the writer just liked some classic Rasslin'.
ReplyDeleteSet theory is imprecise. From the following three distinctions it recognizes only one, namely the first one:
ReplyDelete(1)
The union of the sequence of intervals [0, (n-1)/n] is [0, 1). It is the "set.theoretic" limit.
The *limit* of the sequence of intervals [0, (n-1)/n] is [0, 1]. This is the analytical limit obtained from lim((n-1)/n) = 1.
(2)
The union of the sequence of finite initial segments of the sequence of natural numbers {1}, {1, 2}, {1, 2, 3}, ... has less then aleph_0 elements. This can easily be seen from the figure
1
1, 2
1, 2, 3
...
When shifting all finite rows into the first row, no row with aleph_0 numbers can be created because every row lacks aleph_0 numbers.
The *limit* of the sequence of finite initial segments of the sequence of natural numbers {1}, {1, 2}, {1, 2, 3}, ... has aleph_0 elements.
(3)
A decimal representation (= series or sequence of partial sums like 3.1, 3.14, 3.141, ...) is never irrational. It has aleph_0 rational terms failing to be the limit.
The *limit* of a the sequence can be irrational. Alas it is not subject to the folklore version of Cantor's diagonal argument.
Many defenders of set theory claim that when writing an infinite series like 0.999... automatically the limit 1 is meant by definition. But they do not claim that by the union over {1}, {1, 2}, {1, 2, 3}, ... also automatically the limit is meant. Here they believe that the union has aleph_0 elements. And all formal systems agree to this mistake. Therefore all this is imprecise. No mathematics.
For more information see https://www.hs-augsburg.de/~mueckenh/Transfinity/Transfinity/pdf
Regards, WM
"To infinity, and beyond" = "I can do more than even I think I am capable of doing" This applies to mathematicians as well as the rest of us mere mortals :)
ReplyDeleteYou may be able to do more than you think. But even an infinite sequence of digits shows only an infinity of failures to define a real number precisely. To define an irrational number by fractions (= digits) is absolutely impossible.
DeleteRegards, WM
I agree that the Buzz Lightyear catchphrase was inspired by the "Jupiter and beyond the infinite" title card but I was struck by an epiphany tonight BEFORE I found this blog entry. I believe that Stanley Kubrick created the main title card font design as a foreshadowing of the later one (!). I "fiddled" with the main title card and offer this for consideration. After all, if Stanley Kubrick can "fiddle" with his main title card, I might as well too. :) https://hosting.photobucket.com/images/c385/SSTforumpics/Screenshot_(17721).png
ReplyDelete